An explanatory overview of Ship Grillage Structure

 The hull structure consists of stiffened panels; bottom construction, side shell construction, upper deck construction, bulkhead, etc. Usually stiffened panels consist of plates, beams (small member, secondary member) and girders (big member, primary member). The plate receives loads such as water pressure, the beam supports the loads from the plate and the girder supports the loads from the beam.

A rational grillage structure which has girders crossing each other (mutually-supported structure)  is studied here from a strength viewpoint.

What is a grillage?

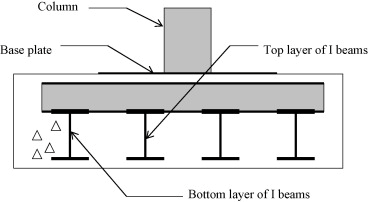
A grillage consists of two layers of “I” beams as shown in Figure. The load from the column is transferred to the base plate and the base plate transfers the load to the concrete. The concrete transfers the load to the top layer of “I” beams and then to the bottom layer of beams. The bottom layer of “I” beams would transfer the load to the concrete below and then to the rock underneath.

When assigning a cargo to a ship or barge, aside from the physical fit of it into the vessel, the cargo weight and centre of gravity must be taken into consideration. When dealing with heavy lift cargo especially, there is a need to ensure that the vessels’ underdeck structure can withstand the loads imposed by the cargo during seagoing conditions.

When the vessels’ internal structure is unable to withstand such loads, bespoke grillages need to be installed to ensure loads are distributed from the cargo support points into the vessels deck structure to avoid damage to either the cargo, the vessel or both.

Grillage Structure

Figure 6.1.1 shows a stiffened panel with length of longer edge a, shorter edge b and uniform load p. It is common sense for the girders to be arranged in the direction of the shorter span as the girders in the longer span are not so effective. Here common sense is proved quantitatively.

As the results for fixed-boundary conditions and for simply supported conditions are similar hereafter, the simply-supported boundary condition is to be applied [1].

The maximum stress s_y is generated at the midpoint O in Fig. 6.1.1 and the stress s_y and the deflection d are as follows:

where d : deflection at point O.

Applying the following notations, maximum stress s_y is described below.

I_x and I_y are sectional moment of inertia with effective breadths

m and n are numbers of girders

e_x and e_y are distances from center of gravity of section to face plates

spaces of girders l_x = b/(m+1) and l_y = a/(n+1)

rigidity ratio per unit breadth ix = I_x/l_x and iy = I_y/l_y

rigidity ratio in longer and shorter edge directions (mutually-supporting ratio)

a = i_x/i_y

Putting weight per unit area of grillage structure as W₁, and weights per unit length of girders in longer and shorter directions as W_x and W_y respectively, the following relation is obtained.

Usually the same scantling girders are to be applied for X and Y directions in a mutually-supported grillage structure. Applying this principle the following results are obtained.

I_x = I_y                Z_x = Z_y             W_x =W_y

In Sect. 1.8, Optimum Design of Beam Section, the relation between the section modulus of a beam and its weight per unit length is explained. This principle can be applied to girders assuming the web thickness is 12 mm. The result is shown in Eq. (6.1.7).

W_y = 1.5 Zy

Where,

W_y : weight per unit length of girder in kgf/m

Z_y : section modulus of girder in cm³

Putting Eqs. (6.1.7) and (6.1.3) into (6.1.4) and with the conditions described by (6.1.5) and (6.1.6), the weight per unit areaW1 of girders is given by the following equation.

Where a grillage (mutually-supported) arrangement is not applied, a girder arrangement in one direction is usually applied. In this case the weight per unit area W₀ is obtained by putting a= 0 in Eq. (6.1.8). The weight ratio W₁/W₀ of the mutually-supported and the one-direction girder arrangement is given as follows:

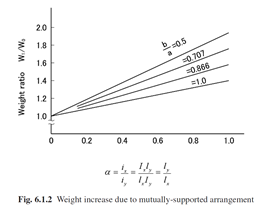
The relation of Eq. (6.1.9) is shown in Fig. 6.1.2 with a along the horizontal axis and b/a as a parameter. It can be seen that the smaller b/a, which means a slender rectangular, and bigger a, mutually-supporting ratio, will bring a bigger weight difference. It is important to note that the ratioW₁/W₀ is always bigger than 1.0 which means the mutually-supported arrangement is always heavier than the one-direction girder arrangement. This result is in agreement with the designer’s common sense.

The stress sy at the point O in Fig. 6.1.1 in the shorter edge direction is expressed by Eq. (6.1.1) and the stress sx at the same point O in the longer edge direction is expressed by the following equations:

s_x/s_y is proportional to the square of b/a which means for a slender panel, s_x is very much smaller than sy and the mutually-supported condition will disappear.

Even in the case of a square panel the one-direction arrangement is better than the mutually-supported arrangement because the weight ratio between two girders with section modulus Zy and one girder with section modulus 2Zy is:

And in Fig. 6.1.2, W₁/W₀ = 1.41 for b/a = 1.0 and a= 1.0 is the same story. Regarding the minimum weight of grillage structure, Yagi and Yasukawa’s study is famous, and Kitamura’s study as a non-linear programming method is also useful.