Natural Frequency of a Simply-supported Beam with a Concentrated Load acting on the Midspan

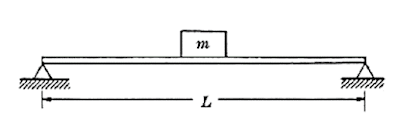
Statement: A simply-supported beam with a concentrated load acting on the midspan is shown in the figure below. If the mass of the beam is negligible compared to the mass acting, find the natural frequency of the system.

Solution:

Let us consider a beam AB of length L is simply supported at A and B as displayed in following figure. Let us thing one load P is acting at the midpoint of the beam.

Reaction force at point A, `R_A` = `\frac{P}{2}`
Reaction force at point B, `R_B` = `\frac{P}{2}`

Let us consider one section XX at a distance x from end support A, let us calculate the bending moment about this section.

`M_x` = `+R_A.x` = `\frac{P}{2}.x` = `\frac{Px}{2}`

The differential equation of elastic curve of a beam is expressed as,
`- M` = `E.I\frac{d^2y}{dx^2}`

By considering the bending moment determined earlier about section XX,
`\Rightarrow E.I\frac{d^2y}{dx^2}` = `-\frac{Px}{2}`

Integrating the above equation,
`E.I\frac{dy}{dx}` = `-\frac{Px^2}{4}+C_1`

Slope will be zero at the centre of the loaded beam. i.e dy/dx = 0, at x = L/2

Hence, `C_1=+\frac{PL^2}{16}`

Therefore, `E.I\frac{dy}{dx}` = `-\frac{Px^2}{4}+\frac{PL^2}{16}` ............................... (i) 

Let us integrate equation (i) and considering the boundary condition, we will have equation for deflection at a section of beam.

`E.I.y` = `-\frac{Px^3}{12}+\frac{PL^2x}{16}+C_2`

Deflection will be zero at support A, x = 0

        `C_2` = 0         

        `E.I.y=-\frac{Px^3}{12}+\frac{PL^2x}{16}` ................................... (ii)

Now, deflections at end support A and B, x = 0,

`y_A=y_B=0`

Deflection will be maximum at the centre of the loaded beam i.e. at x = L/2. Deflection at the centre of the beam, `y_c` could be secured by using the value of x = L/2 in deflection equation (ii),

`E.I.y_c` = `-\frac{PL^3}{96}+\frac{PL^3}{32}` = `\frac{-PL^3+3PL^3}{96}` = `\frac{2PL^3}{96}` = `\frac{PL^3}{48}`

`\therefore y_c` = `\frac{PL^3}{48EI}`

Deflection is also denoted as `\delta`.

Hence, the deflection at mid span of a simply supported beam due to a concentrated load P at the center of the beam is given by,

`\delta=\frac{PL^3}{48EI}`

Where,

            E = Modulus of Elasticity

            I = Moment of Inertia

For small deflections,

`k` = `\frac{P}{\delta}` = `\frac{48EI}{L^3}`

The equation of motion,
`m\ddot{x}+c\dot{x}+kx` =` F(t)`

Here, c = 0 and F(t) =0

Hence, the equation for this free undamped vibration is, `m\ddot{x}+kx` = 0

and, The natural Frequency, `\omega_n=\sqrt{\frac{k}{m}}=\sqrt{\frac{48EI}{mL^3}}`