Abstract
In this experiment, the moment of inertia of flywheel is being studied by variating the point of mass of flywheel. The experiment is conducted by recording the time taken by a fixed load until the point where the load is escaping from the flywheel and the number of rotations done after be independent from the load. The moment of inertia then is calculated by substituting the data obtained from the experiment and the experimental value is calculate and compared to the experimental one.##Are you rushing to make money online? Then this article is for you!
Introduction
Theory
The rotational kinetic energy can be defined by the equation K = 1/2 mv²Where, I is the moment of inertia of the body about the axis of rotation.[2]
Apparatus
1. Flywheel2. Weights
3. Cord
4. Stop-watch
5. Set-square
6. Meter scale
7. Slide calipers
Experimental Data
Table 1: Data for determining the flywheel radius.
|
No. of obs. |
M.S reading |
V.S reading |
Total for the diameter = D (cm) |
Radius D/2 (cm) |
Mean Radius (cm) |
|
1 |
2.4 |
16 |
2.48 |
1.24 |
1.24 |
|
2 |
2.4 |
16 |
2.48 |
1.24 |
|
|
3 |
2.4 |
16 |
2.48 |
1.24 |
Table 2: Data for determining I
|
No . of obj |
Mas s m (gm) |
Height |
No. of revolution |
Mean n1 |
No. of revolu tion |
Mean n2 |
Time t |
Mean time t |
I |
|
Mean I |
|
1 |
500 |
148 |
17 |
15.33 |
43 |
42.33 |
17 |
18.33 |
1764.407 |
|
200360.636 |
|
14 |
42 |
18 |
|||||||||
|
15 |
42 |
20 |
|||||||||
|
2 |
600 |
151 |
15 |
16.33 |
53 |
50.66 |
25 |
22.66 |
|
|
|
|
17 |
51 |
21 |
|||||||||
|
17 |
48 |
22 |
|||||||||
|
3 |
700 |
149 |
17 |
17 |
58 |
58 |
24 |
23 |
|
|
|
|
17 |
58 |
22 |
|||||||||
|
17 |
58 |
23 |
Calculation
Result
The moment of inertia of the flywheel measured about its axis of rotation 200360.636 gm-cm2with an error of 20%.
Discussion
Conclusion
References
[1] https://www.academia.edu[2] http://www.123helpme.com/view.asp?id=149828
[3] Practical physics, Dr. Giasuddin ahmed and Md. Shahabuddin, page- 74
[4] https://blog.oureducation.in/moment-of-inertia-of-a-flywheel-by-falling-weight-method/






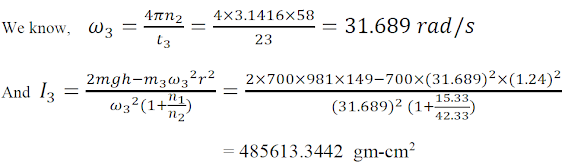
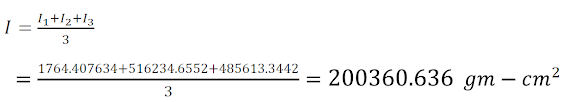

From where you find the standard result.
ردحذفThe standard result was provided by our lab Teacher after the experiment. Then we have checked our error percentage.
حذفOh. Thanks
حذفgood
ردحذف